Mathematicians Are Excited About a Newly Discovered Shape

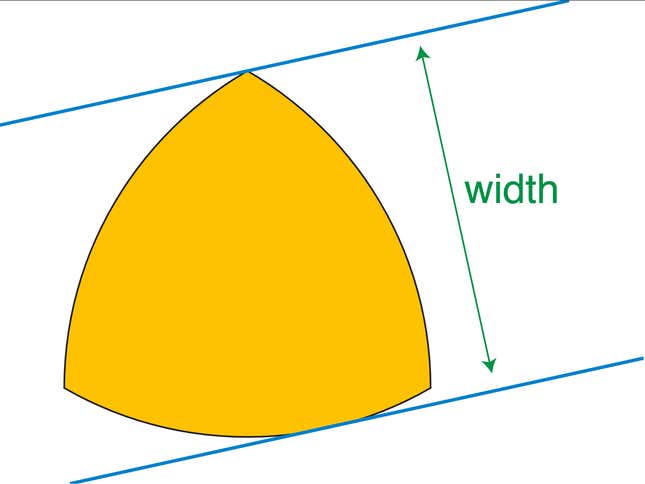
In two dimensions, it’s the Reuleaux triangle: an equilateral triangle with curved arcs connecting each corner, creating a shape with a constant width but a smaller area than a circle. Now, a team of mathematicians say they’ve scaled up the shape into the third dimension and beyond, finding it resolves a math problem that’s been floundering since 1988.

The original problem was put forth by Oded Schramm, a mathematician who considered whether objects of a constant width smaller than a sphere of a higher dimension might exist. The team’s research is currently hosted on the preprint server arXiv.

“The most amazing thing is that volume of each shape is easily computable,” said study co-author Andriy Bondarenko, a mathematician at the Norwegian University of Science and Technology, in an email to Gizmodo. “So we can compare n-volume of the shape with the n-volume of unit ball and see mathematically rigorously that volumes of our shapes are exponentially smaller.”

A Reuleaux triangle (named for a 19th-century engineer, but deployed well before that by scientists like Euler and Leonardo da Vinci) can be formed by constructing three interlocking circles; that space in the middle is the Reuleaux triangle. The Blaschke-Lebesgue theorem, published independently by the respective eponymous mathematicians in 1914 and 1915, stated that the triangle has the least area of all curves of a given constant width. Simply put, that means its width is the same value regardless of where you draw two parallel lines along the shape’s exterior. Get it?

In two dimensions, the shape is a Reuleaux triangle. Seen in three-dimensional space, the shape is oblong, but something our brains can visualize. Beyond the third dimension, the team can mathematically project the the shape’s constant width even in increasing dimensions.

“Perhaps one of the reasons why we succeeded with the construction is that our bodies are in a way ‘unbalanced,’ with lots of volume pushed in a certain direction,” said Andriy Prymark, a mathematician at the University of Manitoba and co-author of the research, in an email to Gizmodo. “In this way, the body is less like a ball, allowing [it] to achieve smaller volume with the same width.”

As reported by New Scientist, at higher dimensions the shape will be proportionally smaller than the sphere of the equivalent dimension. As noted by New Scientist, the shape can roll smoothly like a wheel even though it’s not round.

The shape has yet to have a cool name—consider last year’s discovery of the 13-sided shape called “the hat” and the vampire Einstein (a real label) called “the Spectre.” The new shape has a constant width always smaller than the sphere of its dimension—perhaps “the Svelte?”

More: Upgraded ‘Vampire Einstein’ Shape Finally Solves Vexing Mathematical Pattern Problem